FermCalc - Sugar Calculators

• Chaptalization & Dilution Calculator
• Introduction
• Input Field Definitions
• Output Field Definitions
• Calculation Details
• Mass Balance Equations
• Specify Target SG  >  Calculate H2O/Sugar Additions and Volume
• Specify Target SG and Volume  >  Calculate H2O/Sugar Additions
• Specify H2O/Sugar Additions  >  Calculate Resulting SG and Volume
• High Brix Measurement by Dilution Calculator
• Introduction
• Input Field Definitions
• Output Field Definitions
• Calculation Details

Chaptalization & Dilution Calculator

Introduction

The Chaptalization & Dilution Calculator determines the amounts of sweetener and/or water to add to a given must to achieve a target specific gravity (SG) and volume.  Alternatively, the resulting SG and volume can be calculated from the specified sweetener and water additions.  The sweetener can be either sugar, honey, or concentrate.

FermCalc offers three options for this calculation:

1. Specify Target SG  >  Calculate H2O/Sugar Additions and Volume – Specify the target SG, the initial SG, and the initial volume, and FermCalc will calculate the required additions of water or sweetener as well as the resulting volume after the addition.
2. Specify Target SG and Volume  >  Calculate H2O/Sugar Additions – Specify the target SG, the initial SG, the target volume, and the initial volume, and FermCalc will calculate the required additions of water and/or sweetener to achieve both targets.
3. Specify H2O/Sugar Additions  >  Calculate Resulting SG and Volume – Specify the initial SG, the initial volume, and the amounts of water and/or sugar to be added, and FermCalc will calculate the resulting volume and SG.

If the second calculation option is selected and you get a message that says "Target Volume must be increased or Initial Volume must be reduced.", your target volume is too small to allow the additions that are required to achieve the target specific gravity. In this case, change back to the first calculation option to calculate the minimum target volume required to achieve the desired specific gravity.

The honey and concentrate calculations are very similar. The main difference is that honey additions are specified in mass units, and concentrate additions are specified in volume units.

Input Field Definitions

Sweetener – The type of sweetener to be added, either sugar, honey, or concentrate.  The SG of the sweetener must be specified if honey or concentrate is selected.

Target SG – The desired SG (20°C/20°C) of the must after chaptalization or dilution.  Required only if either the first or second calculation option is selected.  Range: 0.77193 to 1.55454

Initial SG – The SG (20°C/20°C) of the must prior to chaptalization or dilution.  Range: 0.77193 to 1.55454

Target Volume – The desired volume of the must after chaptalization or dilution.  Required only if the second calculation option is selected.

Initial Volume – The volume of the must prior to chaptalization or dilution.  Required only if either the first or third calculation option is selected.

Sugar (or Honey or Concentrate) Added – The amount of sweetener added to the must.  Required only if the third calculation option is selected.

Water Added – The amount of water added to the must.  Required only if the third calculation option is selected.

Output Field Definitions

Sugar (or Honey or Concentrate) Required – The calculated amount of sweetener required to yield the desired specific gravity and volume.  Reported only if either the first or second calculation option is selected.

Water Required – The calculated amount of water required to yield the desired specific gravity and volume.  Reported only if either the first or second calculation option is selected.

Resulting SG – The SG (20°C/20°C) of the must after adding the specified amounts of sweetener and water.  Reported only if the third calculation option is selected.

Resulting Volume – The calculated volume of the must after adding the specified amounts of sweetener and water.  Reported only if the third calculation option is selected.

Calculation Details

Mass Balance Equations

To derive the necessary equations for these calculations we need to perform a simple mass balance. In other words, we need to honor the constraint that the resulting or target mass of any component is equal to the initial mass of the component plus the added mass of that component.  For water and sugar the mass balances are:

mwf = mwi + mwa + mwsa	(1)
msf = msi + mssa	(2)

where:

m_wf = final water mass, kg
m_wi = initial water mass, kg
m_wa = mass of pure water added, kg
m_wsa = mass of water in the sweetener (sugar or honey) added, kg
m_sf = final sugar mass, kg
m_si = initial sugar mass, kg
m_ssa = mass of sugar in the sweetener (sugar or honey) added, kg

We can also write a mass balance on the total mass as:

mtf = mti + msa + mwa

(3)

where:

m_tf = final total mass, kg
m_ti = initial total mass, kg
m_sa = mass of sweetener added, kg

We can relate the masses of the liquids to their volumes and specific gravities as follows:

mwa = vwaρw	(4)
mti = visgiρw	(5)
mtf = vfsgfρw	(6)

where:

v_wa = volume of water added, liters
v_i = initial volume, liters
v_f = final volume, liters
sg_i = initial specific gravity
sg_f = final specific gravity
ρ_w = density of water = 0.9982 kg/liter at 20ºC

If we know the initial and final specific gravities, we can determine initial and final Brix, or percent sugar by weight, from the Brix conversion equation. Knowing Brix we can then relate the initial and final sugar masses to the initial and final total masses as follows:

msi = mtiBi/100	(7)
msf = mtfBf/100	(8)

where:

B_i = initial Brix
B_f = final Brix

Substituting equations (5) and (6) into equations (7) and (8) gives us:

msi = visgiρwBi/100	(9)
msf = vfsgfρwBf/100	(10)

If we're adding honey or concentrate as a sweetener, we'll need to account for the fact that they contain both sugar and water.  We can express the amount of sugar added as:

mssa = msaBs/100

(11)

Where B_s is the Brix of the sweetener.

Substituting equations (9) through (11) into equation (2) we get the sugar mass balance equation:

vfsgfρwBf = visgiρwBi + msaBs

(12)

And substituting equations (4) through (6) into equation (3) we get the total mass balance equation:

vfsgfρw = visgiρw + msa + vwaρw

(13)

Equations (12) and (13) form the basis for all of these calculations.  They'll just be re-arranged and solved differently depending on what we're solving for.  There are three calculation options to consider.

1. Specify Target SG  >  Calculate H2O/Sugar Additions and Volume
2. Specify Target SG and Volume  >  Calculate H2O/Sugar Additions
3. Specify H2O/Sugar Additions  >  Calculate Resulting SG and Volume

Option 1: Specify Target SG  >  Calculate H2O/Sugar Additions and Volume

In this case the known values are v_i, sg_i, and sg_f.

If the target gravity is greater than the initial gravity, we know we'll be adding a sweetener and no water, so equation (13) reduces to:

vfsgfρw = visgiρw + msa

(14)

Substituting the right-hand side of equation (14) into equation (12) we get:

(visgiρw  + msa)Bf = visgiρwBi  + msaBs

(15)

Then we can re-arrange equation (15) to solve for the amount of sweetener to add:

msa = visgiρw(Bf - Bi) / (Bs - Bf)

(16)

In the case of concentrate, the mass calculated from equation (23) is converted to a volume as:

vca = msa / (sgcρw)

(17)

where:

v_ca = volume of concentrate added, liters
sg_c = concentrate specific gravity

Knowing how much sweetener we're adding we can then re-arrange equation (14) to solve for the final volume after the addition of the sweetener, which gives us:

vf = (visgiρw + msa) / (sgfρw)

(18)

The graphs below validate equations (16) and (18) by comparing their results to tabular data from various sources and to the results of my own experiments.

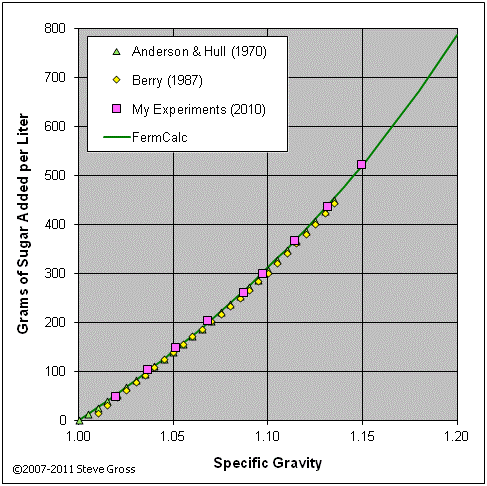
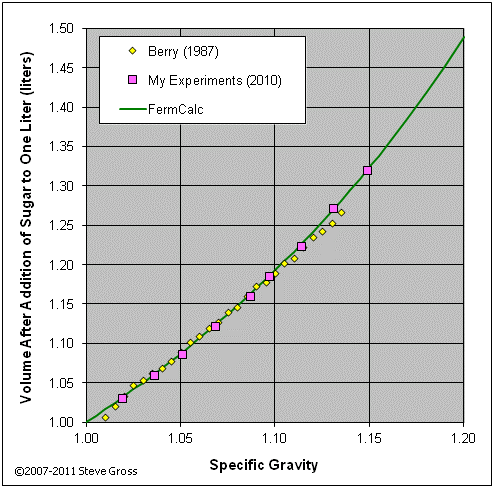
If the target gravity is less than the initial gravity, we know we'll need to add water to reduce the gravity. Since we know we'll be adding water only and no sugar, equation (12) reduces to:

vfsgfρwBf = visgiρwBi

(19)

Now we can solve equation (19) for the final volume:

vf = (visgiBi) / (sgfBf)

(20)

Since we know we'll only be adding water to the mixture, equation (13) reduces to:

vfsgfρw = visgiρw + vwaρw

(21)

Solving equation (21) for the volume of water to add we get:

vwa = vfsgf - visgi

(22)

Option 2: Specify Target SG and Volume  >  Calculate H2O/Sugar Additions

In this case the known values are v_i, v_f, sg_i, and sg_f. To calculate the amount of sugar to add, we simply need to re-arrange equation (12) and solve for the amount of sweetener to add, or:

msa = (vfsgf ρwBf - visgiρwBi) / Bs

(23)

In the case of concentrate, the mass calculated by equation (23) is converted to a volume using equation (17).   Knowing the amount of sweetener to add we can then re-arrange equation (13) to solve for the amount of water to add:

vwa = (vfsgfρw - visgiρw - msa) / ρw

(24)

The graph below validates equation (23) by comparing its results to tabular data from various sources and to the results of my own experiments.

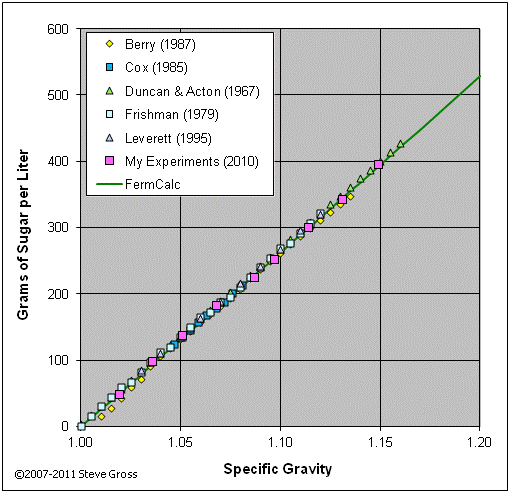
Option 3: Specify H2O/Sugar Additions  >  Calculate Resulting SG and Volume

In this case the known values are v_i, sg_i, m_sa, and m_wa. To calculate the resulting specific gravity sg_f we first substitute the right-hand side of equation (13) into equation (12) to get:

(visgiρw + msa + vwaρw)Bf = visgiρwBi + msaBs

(25)

Then solving for B_f we get:

Bf = (visgiρwBi + msaBs) / (visgiρw + msa + vwaρw)

(26)

Knowing B_f we can then determine sg_f from the Brix conversion equation.

After we determine sg_f we can solve equation (13) for v_f to get:

vf = (visgiρw + msa + vwaρw) / (sgfρw)

(27)

High Brix Measurement by Dilution Calculator

Introduction

The High Brix Measurement by Dilution Calculator determines the specific gravity of a liquid from a specific gravity measurement after it has been diluted with a given volume of water.  This calculation is useful for determining the specific gravity of high-Brix liquids such as honey or concentrates with specific gravities that are outside of the range of standard hydrometers or refractometers.

The calculator will adjust the hydrometer reading for temperature if the measurement temperature and the hydrometer calibration temperature are provided.  If the entered SG value is already corrected for temperature, or if temperature corrections are not desired, simply enter 20°C (68°F) in both of the temperature fields and no corrections will be made.  Details of the hydrometer temperature correction calculations can be found here.  The temperature correction is applied only to the hydrometer reading and not to the liquid volumes.  The temperatures of the original liquid and the added water should be about the same so that the correct dilution ratio is used in the calculation.

The accuracy of this calculation is highly dependent on how thoroughly the diluted sample is mixed.  If the diluted sample is not mixed well, the measured SG might not be representative of the mixture, which would result in an inaccurate SG estimate for the original liquid.

Input Field Definitions

Measurement Type – Select from hydrometer or refractometer.  Hydrometer measurements will be temperature corrected if desired.

Sample Volume – The original volume of the sample to be diluted.

Water Added – The volume of water added to dilute the sample.

Refractometer Reading – The refractometer reading for the diluted sample.  Required only if refractometer was selected as the measurement type.  Range: 0° to 100°Brix

Hydrometer SG Reading – The hydrometer SG reading for the diluted sample.  Required only if hydrometer was selected as the measurement type.  Range: 0.77193 to 1.55454

SG Reading Temperature – The temperature of the diluted sample at the time of the SG reading.  Required only if hydrometer was selected as the measurement type.  Range: 0°C (32°F) to 40°C (104°F)

Calibration Temperature – The hydrometer calibration temperature of the hydrometer used to take SG reading.  Required only if hydrometer was selected as the measurement type.  Range: 0°C (32°F) to 40°C (104°F)

Output Field Definitions

Corrected SG (20°C/20°C) – The temperature-corrected SG value.  Reported only if hydrometer was selected as the measurement type.

Calculated Sample SG – The calculated SG of the original sample prior to dilution.

Diluted Volume – The calculated volume of the diluted sample, which is not simply the sum of the sample and water volumes because of solution effects.

Calculation Details

We can calculate the specific gravity of the original sample using the mass balance equations derived above.  In this case the known values are v_i, sg_f, B_f, and m_wa.  Given that we are adding only water and no sugar, we can re-arrange equation (13) to solve for the final (diluted) volume v_f to get:

vf = (visgi + vwa) / sgf

(28)

Then we can solve equation (12) for the original Brix of the sample B_i to get:

Bi = (vfsgfBf) / (visgi)

(29)

Since sg_i and B_i are interdependent, the solution must be obtained iteratively.

© 2007-2021 Steve Gross
Last updated 11 September 2021.